The Risk-Constrained Kelly Criterion: From the Foundations to Trading – Part I

The Kelly Criterion is good enough for long-term trading where the investor is risk-neutral and can handle big drawdowns. However, we cannot accept long-duration and big drawdowns in real trading. To overcome the big drawdowns caused by the Kelly Criterion, Busseti et al. (2016) offered a risk-constrained Kelly Criterion that incorporates maximizing the long-term log-growth rate together with the drawdown as a constraint. This constraint allows us to have a smoother equity curve. You will learn everything about the new type of Kelly Criterion here and apply a trading strategy to it.

This blog covers:

• The Kelly criterion
• The risk-constrained Kelly criterion
• A trading strategy based on the risk-constrained Kelly Criterion

The Kelly criterion

The Kelly Criterion is a well-known formula for allocating resources into a portfolio.

You can learn more about it by using many resources on the Internet. For example, you can find a quick definition of Kelly Criterion, a blog with an example of position sizing, and even a webinar on Risk Management.

We won’t go deep on the explanation since the above links already do that. Here, we provide the formula and some basic explanation for using it.

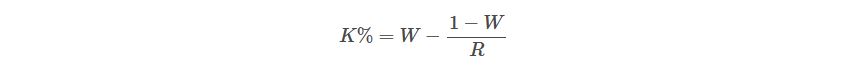
here,

• K% = The Kelly percentage
• W = Winning probability
• R = Win/loss ratio

Let’s understand how to apply.

Suppose we have your strategy returns for the past 100 days. We get the hit ratio of those strategy returns and set it as “W”. Then we get the absolute value of the mean positive return divided by the mean negative return. The resulting K% will be the fraction of your capital for your next trade.

The Kelly Criterion ensures the maximum long-term return for your trading strategy. This is from a theoretical perspective. In practice, if you applied the criterion in your trading strategy, you would face many long-lasting big drawdowns.

To solve this problem, Busseti et al. (2016) provided the “risk-constrained Kelly Criterion”, which allows us to have a smoother equity curve with less frequent and small drawdowns.

The risk-constrained Kelly criterion

The Kelly criterion relates to an optimization problem. For the risk-constraint version, we add, as the name says, a constraint. The basic principle of the constraint can be formulated as:

The drawdown risk is defined as Prob(Minimum Wealth < alpha), where alpha ∈ (0, 1) is a given target (undesired) minimum wealth. This risk depends on the bet vector b in a very complicated way. The constraint limits the probability of a drop in wealth to value alpha to be no more than beta.

The authors highlight the important issue that the optimization problem with this constraint is highly complex thing to solve. Consequently, to make it easier to solve it, Busseti et al. (2016) provided a simpler optimization problem in case we have only 2 outcomes (win and loss), which is the following:

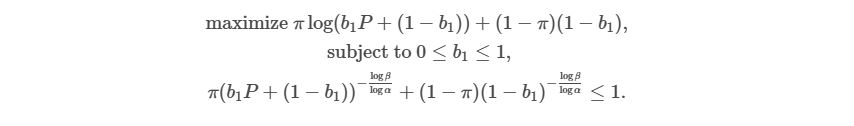
Where:

Pi: Winning probability

P: The payoff of the win case.

b1: The kelly fraction to be found. b1= K%. The control variable of the maximization problem

Lambda: The risk aversion of the trader: log(beta)/log(alpha)

Please take into account that the win/loss ratio defined in the basic criterion named as R is:

R = P – 1, where P is the payoff of the win case described for the risk-constrained Kelly criterion.

You might ask now: I don’t know how to solve that optimization problem! Oh no!

I can surely help with that! The authors have proposed a solution. See below!

The solution algorithm for the risk-constrained Kelly criterion goes like this:

If B1 = (pi*P-1)/(P-1) satisfies the risk constraint, then that is the solution. Otherwise, we find b1 by finding the b1 value for which

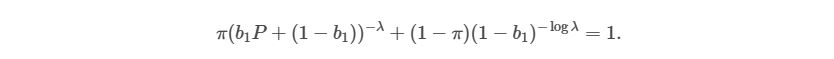
As explained by the authors, the solution can be found with a bisection algorithm.

Stay tuned for Part II for a sample trading strategy based on the risk-constrained Kelly Criterion.

Originally posted on QuantInsti blog.